JEE 2012 Problem & Solution

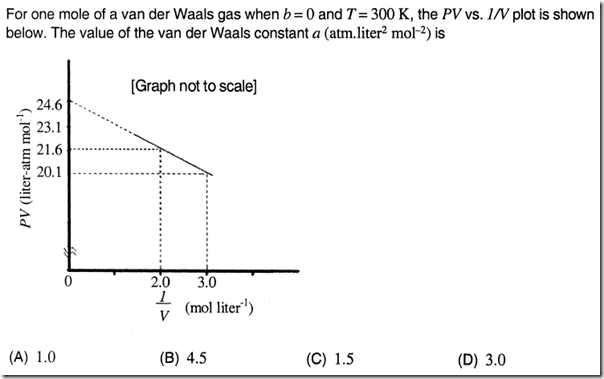
Solution

We have, $\left( {P + \frac{{{n^2}a}}{{{V^2}}}} \right)\left( {V - nb} \right) = nRT$

As per the question, $\left( {P + \frac{a}{{{V^2}}}} \right)V = RT$

$\Rightarrow PV + \frac{a}{V} = RT$

$\Rightarrow PV = - a\frac{1}{V} + RT$

Since PV is the y-axis and 1/V is the x-axis, slope=-a.

$Slope = \frac{{20.1 - 21.6}}{{3.0 - 2.0}} = - 1.5 = - a$

Thus,a = 1.5 .

(C)

Manish Verma Education